December 4, 2024 by Intelligent Computing
Collected at: https://phys.org/news/2024-12-path-topology-quantum.html
Can insights from topology—the study of the properties of 3D objects that persist when an object is stretched or compressed—be applied in the field of quantum information processing? Juan Lin, Shou-Bang Yang, Fan Wu, and Zhen-Biao Yang, researchers at Fuzhou University, China, believe so.
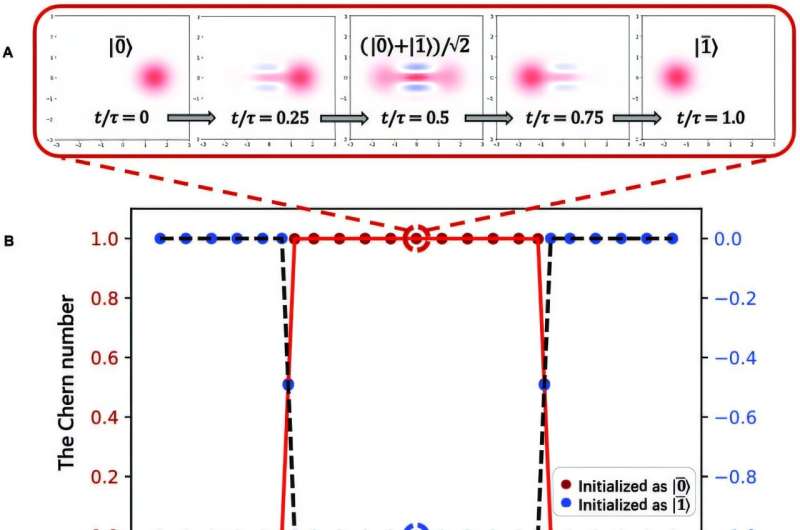
Accordingly, they studied changes in the first Chern number, a topological invariant, in connection with a Kerr nonlinear oscillator. Their research was published August 5 in Intelligent Computing in an article titled “Topological Transitions in a Kerr Nonlinear Oscillator.”
It is important to identify the topological transition in a quantum system. This transition is signaled by a discrete jump in the first Chern number. In Lin, Yang, Wu, and Yang’s method, this Chern number is computed by means of the relevant Berry curvature—a representation of the properties of the parameters of a system—and a polar angle obtained by measuring 3 physical observables.
This method for the observation of topological transition in a controlled Kerr nonlinear oscillator system is aligned with the concept of quantum simulation first proposed by physicist Richard Feynman in his 1982 article “Simulating Physics with Computers.”
Previous studies observed topological transformations by means of the first Chern number and Berry curvature, but did not use a Kerr nonlinear oscillator. A Kerr nonlinear oscillator can be used as a qubit in quantum computing, as it can generate two simultaneous opposite states.
Using a Kerr nonlinear oscillator increases the required operation time, but unlike other methods, can encode continuous variables. Lin, Yang, Wu, and Yang were able to mitigate the increase in required operation time using a “shortcut to adiabaticity” protocol.
Although so far the results have been investigated within a single Kerr nonlinear oscillator, the method can be extended to two or more interaction-involved topological properties in continuous variable systems.
More information: Juan Lin et al, Topological Transitions in a Kerr Nonlinear Oscillator, Intelligent Computing (2024). DOI: 10.34133/icomputing.0099

Leave a Reply